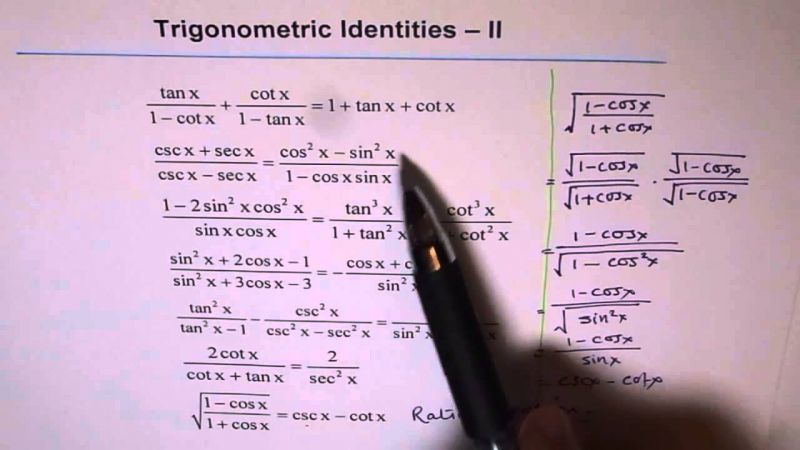
Instead, we have to use logical steps to show that one side of the equation can be transformed to the other side of the equation. Sometimes, we will work separately on each side, till they meet in the middle.
ESSENTIAL IDENTITIES:
sin(x)=1csc(x)
cos(x)=1sec(x)
tan(x)=1cot(x)
sec(x)=1cos(x)
csc(x)=1sin(x)
cot(x)=1tan(x)
tan(x)=sin(x)cos(x)
sin(−x)=−sin(x)
cos(−x)=cos(x)
tan(−x)=−tan(x)
sin2(x)+cos2(x)=1
1+tan2(x)=sec2(x)
1+cot2(x)=csc2(x)
sin(a+b)=sin(a)cos(b)+cos(a)sin(b)
sin(a−b)=sin(a)cos(b)−cos(a)sin(b)
cos(a+b)=cos(a)cos(b)−sin(a)sin(b)
cos(a−b)=cos(a)cos(b)+sin(a)sin(b)
tan(a+b)=tan(a)+tan(b)1−tan(a)tan(b)
tan(a−b)=tan(a)−tan(b)1+tan(a)tan(b)
sin(x)+sin(y)=2sin(x+y2)cos(x−y2)
sin(x)−sin(y)=2cos(x+y2)sin(x−y2)
cos(x)+cos(y)=2cos(x+y2)cos(x−y2)
cos(x)−cos(y)=−2sin(x+y2)sin(x−y2)
sin(2x)=2sin(x)cos(x)
cos(2x)=cos2(x)−sin2(x)=1−2sin2(x)=2cos2(x)−1
sin(x2)=±1−cos(x)2−−−−−−√
cos(x2)=±1+cos(x)2−−−−−−√
tan(x2)=±1−cos(x)1+cos(x)−−−−−−√=1−cos(x)sin(x)=sin(x)1+cos(x)
sin(x)cos(y)=sin(x+y)+sin(x−y)2
cos(x)cos(y)=cos(x+y)+cos(x−y)2
sin(x)sin(y)=cos(x−y)−cos(x+y)2
Also Read
Self Decode: We uproot your hidden diseases!
Planets consisting of Volcanoes with Hydrogen element may bear life
Tata motors to step on race track with its Geneva showcase sports car